Better IOL calculation in post-LASIK eyes
To heighten precision, use total keratometry, not imaginary numbers
In our cover story, Dr Giacomo Savini explains how total keratometry (TK) can improve precision in IOL calculation. Image credit: ©untitledtitle – stock.adobe.com
There is always an element of jeopardy when performing the work required to select the right IOL for the patient. Small errors in biometry or transcription errors can result in large refractive surprises. Then there is a wide range of formulae to choose from to calculate the right IOL for the patient, and sometimes it needs a great deal of care, judgement, and experience to select the right lens for each patient. This process becomes far more complicated in post–laser in situ keratomileusis (LASIK) and post–photorefractive keratectomy (PRK; postexcimer) corneas.1,2 As we will discover, certain IOL power equations like Haigis are simply not appropriate for use in postexcimer corneas, and others have to make further assumptions and additional calculations, which can further increase the risk of introducing an error, to account for the fact that these corneas have had their shape and refractive power changed by previous laser surgery.
Figure 1: Keratometric index error. Preoperatively (top), a fixed ratio between the anterior and posterior corneal curvature allows the calculation of the corneal power with the fictitious 1.3375 (or 1.3315) keratometric index. Postoperatively, the anterior corneal surface is flattened, the ratio is altered and the keratometric index leads to a wrong calculation of the corneal power, which is overestimated.
Assumptions and errors
There are 3 main sources of IOL power calculation error.3
First, and most importantly, is the keratometric index error. To calculate the dioptric focusing power of the cornea, you need to know both the anterior and posterior corneal radii (Figure 1).
Most corneal topographers and ocular biometers measure only the anterior radius. The keratometric index, which is 1.3375 in most devices, is used to calculate the dioptric power of the whole cornea from just the anterior corneal curvature and thus accounts for the negative power introduced by the posterior corneal surface (Figure 1).4 This has some big advantages: Total corneal power can be calculated without needing to measure the posterior corneal curvature. But the keratometric index comes with a big disadvantage: It is a completely fictitious number assuming that the relationship between the anterior and posterior radii is fixed. The relationship it relies on completely breaks down in postexcimer corneas, where the anterior to posterior ratio is disrupted by the laser. To solve this problem, the keratometric index should be adjusted according to the amount of laser induced correction,5 a method that has been found to be accurate but requires knowledge of the surgical treatment, which is often unavailable.
There is also the radius measurement error. This occurs when the optical zone created by the laser is small or decentered, so that the corneal radius is not measured along the visual axis but in an area where the corneal curvature is likely to be different (usually steeper). This error is unusual in eyes that underwent excimer laser surgery in the past 20 years and is more common when the refractive error was corrected with older lasers in the 1990s.
The last source of miscalculations is the “formula error,” where formulae like Hoffer Q,6 Holladay 1,7 and SRK/T8 use corneal power to predict the effective lens position (ELP). Because corneal power is changed by the excimer laser, using its postoperative value leads to a wrong estimation of the ELP. This error can be solved using the Double-K method,9 where the pre-excimer laser keratometry (if available) is used to estimate the ELP, and postoperative keratometry is used to calculate the vergence of rays. Formulae like Haigis’,10 which does not predict the ELP from keratometry, are not affected by this error.
Figure 2: Overview of how total keratometry (TK) values are generated. A single instrument performs telecentric keratometry and optical coherence tomography (OCT) imaging of the cornea and is processed to generate TK values that are plugged in directly to subsequent IOL power calculation formulae.
Better biometry brings reality into the equation
Given that modern ocular biometry instruments include optical coherence tomography (OCT) imaging of the cornea, we are now at a stage where certain instruments can make direct measurements of both the anterior and posterior corneal curvature, as well as pachymetry of the entirety of the cornea, in addition to the usual measurements of axial length, anterior chamber depth, lens thickness, and central corneal thickness. Rather than using the imaginary number, perhaps we could use these measurements to generate more accurate total corneal power values? We can, and the combination of these measurements is called total keratometry (TK) (Figure 2), and it is in effect equivalent to K readings taken in unoperated eyes. This means TK values can be plugged into existing IOL power equations where K values were used before, whereas existing optimised IOL constants (such as User Group for Laser Interference Biometry and IOLCon.org constants) can still be used.
Figure 3: Proportion of post-excimer laser surgery eyes with mean absolute errors of 0.5, 1.0 and 2.0 D achieved with different IOL power calculation methods, as reported by Wang et al in 2019.11
TK in clinical practice
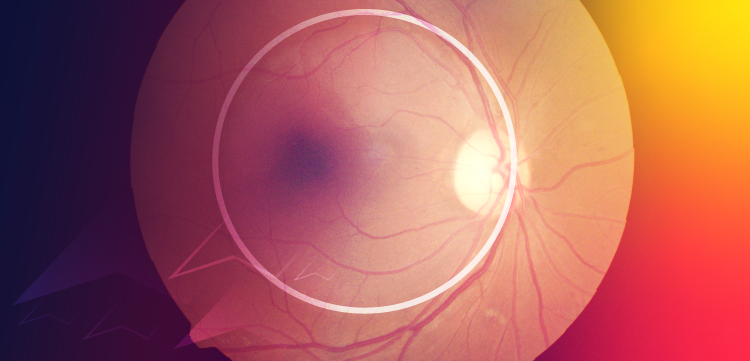
TK can be entered into the Haigis formula. This combination has been shown to work well in eyes that have previously undergone myopic LASIK.9 In addition, this combination has worked better than not only standard Haigis but also 2 formulae designed for use in postexcimer corneas, Haigis L and Barrett True K, all without having to take historical refraction data into account (Figure 3).11
This is understandable: The Haigis formula does use K values to predict the ELP, as it instead uses anterior chamber depth and axial length—and this has also been my experience. By substituting the imaginary keratometric index for total TK obtained from an IOLMaster 700 (Carl Zeiss Meditec AG; Jena, Germany), I have been able to use the Haigis formula with ULIB constants to select the appropriate IOL in a population of 23 post–refractive surgery patients (prior myopic LASIK or PRK) who underwent cataract surgery, with excellent outcomes that are better than those achieved with Barrett True K and Haigis L. Over half of these patients had a prediction error within ±0.25 D, with 78.3% and 91.3% being within ±0.50 D and ±1.00 D, respectively (Figure 4).
Figure 4: Proportion of eyes that were within 0.25, 0.5, 1 and 2 D of target postoperative refraction when total keratometry (TK) values were used in the Haigis formula.
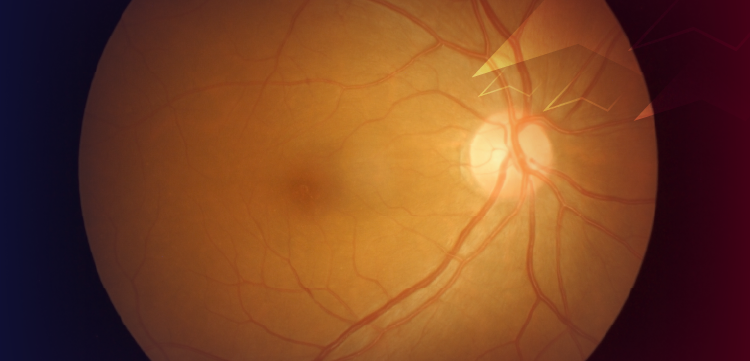
An example of a patient, where TK and Haigis were used to predict postoperative refractive outcomes in a patient, is presented in Figure 5. We selected a 19.5-D IOL and ended up with postoperative refraction of –0.25 D and a prediction error of –0.12 D.
Conclusion
IOL power calculation is a complex and challenging process to consistently get right, particularly in postexcimer patients, so anything that keeps the process simple and minimises the opportunity for errors to be introduced is valuable to surgeons. By exploiting the capabilities of modern ocular biometers that include advanced OCT imaging components that can accurately characterise the shape and thickness of the cornea, we can generate TK measurements that can simply, directly, and automatically be entered into IOL calculation formulae within the instrument.
Figure 5: Example of a post-LASIK patient who underwent cataract surgery and received a 19.5 D IOL with a postoperative refraction of0.25 D and a prediction error of0.12 D according to the combination of TK and Haigis formula. Panel (A) shows the IOLMaster 700 report for the eye using TK values (as if the patient had not previously had laser vision correction) using Haigis, and panel (B) shows the Barrett TK True K and Haigis L report where the patient was entered as a post-LASIK patient.
This eliminates a stage where transcription errors might occur; no external websites need to be used during IOL power calculation. TK enables us to use the Haigis formula in postexcimer eyes as if the eyes were unoperated on, and this eliminates the need to use more complex formulae that require additional measurements, and the results we have seen to date have been at least as good as these formulae.
My own experience, and that of many of my peers, is that the Haigis formula, in combination with TK, delivers more accurate results than Barrett True K or Haigis L in postexcimer corneas, in a simpler and easier manner.
References
1. Savini G, Hoffer KJ. Intraocular lens power calculation in eyes with previous corneal refractive surgery. Eye Vis (Lond). 2018;5:18. doi:10.1186/s4066201801105.
2. Alio JL, Abdelghany AA, Abdou AA, Maldonado MJ. Cataract surgery on the previous corneal refractive surgery patient. Surv Ophthalmol. 2016;61(6):769777. doi:10.1016/j.survophthal.2016.07.001.
3. Hoffer KJ. Intraocular lens power calculation after previous laser refractive surgery. J Cataract Refract Surg. 2009;35(4):759765. doi:10.1016/j.jcrs.2009.01.005.
4. Seitz B, Langenbucher A, Nguyen NX, Kus MM, Küchle M. Underestimation of intraocular lens power for cataract surgery after myopic photorefractive keratectomy. Ophthalmology. 1999;106(4):693-702. doi:10.1016/S0161-6420(99)90153-7
5. Savini G, Barboni P, Zanini M. Correlation between attempted correction and keratometric refractive index of the cornea after myopic excimer laser surgery. J Refract Surg. 2007;23(5):461-466. doi:10.3928/1081-597X-20070501-07
6. Hoffer KJ. The Hoffer Q formula: A comparison of theoretic and regression formulas. J. Cataract Refractive Surg. 1993;19(6):700-712. doi:10.1016/s0886-3350(13)80338-0
7. Holladay JT, Prager TC, Chandler TY, Musgrove KH, Lewis JW, Ruiz RS. A three-part system for refining intraocular lens power calculations. J Cataract Refract Surg. 1988;14(1):17-24. doi:10.1016/s0886-3350(88)80059-2
8. Retzlaff JA, Sanders DR, Kraff MC. Development of the SRK/T intraocular lens implant power calculation formula. J. Cataract Refractive Surg. 1990;16(3):333-340. doi:10.1016/s0886-3350(13)80705-5
9. Aramberri J. Intraocular lens power calculation after corneal refractive surgery: double-K method. J Cataract Refract Surg. 2003;29(11):2063-2068. doi:10.1016/s0886-3350(03)00957-x
10. Haigis W, Lege B, Miller N, Schneider B. Comparison of immersion ultrasound biometry and partial coherence interferometry for intraocular lens calculation according to Haigis. Graefes Arch Clin Exp Ophthalmol. 2000;238(9):765-773. doi:10.1007/s004170000188
11. Wang L, Spektor T, de Souza RG, Koch DD. Evaluation of total keratometry and its accuracy for intraocular lens power calculation in eyes after corneal refractive surgery. J Cataract Refract Surg. 2019;45(10):1416-1421. doi:10.1016/j.jcrs.2019.05.020
All images courtesy of Giacomo Savini, MD
